Calculating tidal accelerations on Earth due to other solar system planets, and then on Europa due to Jupiter for comparison.
Following the material in section 2.3.3 of Lowrie's Fundamentals of Geophysics (highly recommended undergrad or intro graduate geophysics textbook), we can calculate the tidal accelerations on the Earth due to various other bodies in the solar system and compare them in a Matlab script called tidalaccel.m for the purpose. How strong are the pulls of other planets compared to that of the Sun or Moon? Will the other planets have any noticeable effect on Earth's tides? Then we can also calculate the tidal acceleration on Europa due to Jupiter and compare that to the Earth ones to get a feel for the stresses seen in Europa's cracking ice.
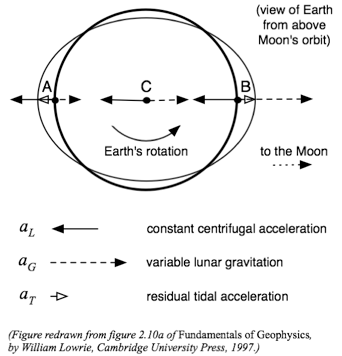
The figure demonstrates the tidal bulge of the Earth due to the combination of the Moon's direct gravitational pull and the centrifugal force in the Earth's and Moon's revolution about their center of mass (which happens to be inside the Earth, but not at its center). Earth's rotation on its axis is ignored here - when the Earth rotates under this bulge we see the twice-daily tidal maxima as points on the Earth surface rotate under the bulges, but here we will only calculate and compare the tidal acceleration causing the bulge itself. If we neglect the Earth's rotation, then as the Earth revolves about the Earth/Moon center of mass, all points in the Earth circumscribe the same size circle. This means that all points in the Earth experience the same centrifugal acceleration from this revolution. In this scenario, at any given time in the revolution the centrifugal acceleration leads away from the Moon while the Moon's gravitational attraction pulls toward the Moon, as we see in the figure. Since the strength of the gravitational acceleration from the Moon's attraction is distance dependent (falls off as 1/r2), it doesn't balance equally with the centrifugal acceleration everywhere; the difference between the two gives the tidal acceleration. At the center of the Earth (point C) the two are equal and there is no residual tidal acceleration. On the Earth's surface closer to the Moon (point B) the gravitational attraction is stronger so the tidal acceleration points toward the Moon. On the far surface (point A), the gravitational acceleration is much less so the tidal acceleration points away from the Moon. If one considers the Earth's rotation on its axis in this scenario after all, one finds subtleties like the bulge not pointing exactly toward and away from the Moon, leading to higher order (smaller) effects, but again, let us ignore that and just compare the main accelerations here.
Adding Newton's gravitation equation to the above leads to the following equations for the tidal acceleration at points A & B on the surface of the Earth caused by the Moon, or any other remote body for that matter (Lowrie's equations 2.28 & 2.30).
Tidal acceleration at point A (eqn 2.30):
Tidal acceleration at point B (eqn 2.28):
In these equations, aT is the tidal acceleration, G is the gravitational constant, m is the mass of the remote body tugging on the Earth, R is the radius of the Earth, and rL is the separation between the Earth and the body. These accelerations at A and B are very close to each other, and in fact Lowrie shows they are equal to first order. In any case, what we really find when calculating these accelerations due to various solar system bodies is the wide range in order of magnitude of the accelerations. The farther an object is away, the very much less effect it has, because the tidal acceleration to first order falls off as 1/r3.
This doesn't have to be just for the Earth and Moon, we can stick anything in there. So let's run this Matlab script which implements these equations on different bodies in the solar system. First we compute the tidal accelerations on Earth from the Sun, Moon, and other planets. Then we compute the tidal acceleration on Europa from Jupiter.
We see that the tidal pull from the Moon is the strongest, followed by the Sun which has half the effect of the Moon - it's very much larger than the Moon but farther away, and tidal acceleration falls off strongly with distance. The rest of the planets' effects are infinitesimal in comparison; note the next strongest effect after the Sun's is the tidal acceleration due to Venus, which is 10000 times smaller.
In contrast, notice that the tides on Europa are 1000 times stronger than those here on Earth, since Jupiter is both very massive and very close to Europa. (Those on Io are even stronger.) That's a lot of energy being pumped into Europa via tides!
Again, the Matlab script for this is available here.
The figure demonstrates the tidal bulge of the Earth due to the combination of the Moon's direct gravitational pull and the centrifugal force in the Earth's and Moon's revolution about their center of mass (which happens to be inside the Earth, but not at its center). Earth's rotation on its axis is ignored here - when the Earth rotates under this bulge we see the twice-daily tidal maxima as points on the Earth surface rotate under the bulges, but here we will only calculate and compare the tidal acceleration causing the bulge itself. If we neglect the Earth's rotation, then as the Earth revolves about the Earth/Moon center of mass, all points in the Earth circumscribe the same size circle. This means that all points in the Earth experience the same centrifugal acceleration from this revolution. In this scenario, at any given time in the revolution the centrifugal acceleration leads away from the Moon while the Moon's gravitational attraction pulls toward the Moon, as we see in the figure. Since the strength of the gravitational acceleration from the Moon's attraction is distance dependent (falls off as 1/r2), it doesn't balance equally with the centrifugal acceleration everywhere; the difference between the two gives the tidal acceleration. At the center of the Earth (point C) the two are equal and there is no residual tidal acceleration. On the Earth's surface closer to the Moon (point B) the gravitational attraction is stronger so the tidal acceleration points toward the Moon. On the far surface (point A), the gravitational acceleration is much less so the tidal acceleration points away from the Moon. If one considers the Earth's rotation on its axis in this scenario after all, one finds subtleties like the bulge not pointing exactly toward and away from the Moon, leading to higher order (smaller) effects, but again, let us ignore that and just compare the main accelerations here.
Adding Newton's gravitation equation to the above leads to the following equations for the tidal acceleration at points A & B on the surface of the Earth caused by the Moon, or any other remote body for that matter (Lowrie's equations 2.28 & 2.30).
Tidal acceleration at point A (eqn 2.30):
Tidal acceleration at point B (eqn 2.28):
In these equations, aT is the tidal acceleration, G is the gravitational constant, m is the mass of the remote body tugging on the Earth, R is the radius of the Earth, and rL is the separation between the Earth and the body. These accelerations at A and B are very close to each other, and in fact Lowrie shows they are equal to first order. In any case, what we really find when calculating these accelerations due to various solar system bodies is the wide range in order of magnitude of the accelerations. The farther an object is away, the very much less effect it has, because the tidal acceleration to first order falls off as 1/r3.
This doesn't have to be just for the Earth and Moon, we can stick anything in there. So let's run this Matlab script which implements these equations on different bodies in the solar system. First we compute the tidal accelerations on Earth from the Sun, Moon, and other planets. Then we compute the tidal acceleration on Europa from Jupiter.
| Tidal accels (m/s^2) at pts A & B on Earth due to solar system bodies. | ||
| due to | a_T at A | a_T at B |
|---|---|---|
| Sun | 5.05392e-07 | 5.05456e-07 |
| Moon | 1.09338e-06 | 1.14948e-06 |
| Mercury | 3.65155e-13 | 3.65232e-13 |
| Venus | 5.80684e-11 | 5.80952e-11 |
| Mars | 1.98055e-12 | 1.98103e-12 |
| Jupiter | 6.49978e-12 | 6.49998e-12 |
| Saturn | 2.31856e-13 | 2.31859e-13 |
| Uranus | 3.63353e-15 | 3.63356e-15 |
| Neptune | 1.05777e-15 | 1.05778e-15 |
| Pluto | 5.57134e-20 | 5.57136e-20 |
| Tidal accel (m/s^2) at pts A & B on Europa due to Jupiter. | ||
| due to | a_T at A | a_T at B |
|---|---|---|
| Jupiter | 0.00131186 | 0.00132109 |
We see that the tidal pull from the Moon is the strongest, followed by the Sun which has half the effect of the Moon - it's very much larger than the Moon but farther away, and tidal acceleration falls off strongly with distance. The rest of the planets' effects are infinitesimal in comparison; note the next strongest effect after the Sun's is the tidal acceleration due to Venus, which is 10000 times smaller.
In contrast, notice that the tides on Europa are 1000 times stronger than those here on Earth, since Jupiter is both very massive and very close to Europa. (Those on Io are even stronger.) That's a lot of energy being pumped into Europa via tides!
Again, the Matlab script for this is available here.